CRACK WIDTH CALCULATIONS ACCORDING TO EUROCODE 2. Eurocode 2 (EN1992-1-1) proposes equations for the calculation of crack width, taking into account several parameters, like the concrete and steel strain and reinforcing bar diameters. According to Eurocode 2, the crack width, wk, can be calculated from the following equation: wk = sr,max (εsm –εcm). Prestressed Concrete Structures. This feature is not available right now. Please try again later. The flexural crack width expression in the above equation, with, is used in ACI 318-95 in the following form: (8) A maximum value of z = 3064.5 N/mm is permitted for interior exposure, corresponding to a limiting crack width of 0.41 mm. ACI 318-95 also limits the value of z.
Crack Width Calculation (Negative Crack Width Determination)
Crack Width Calculation (Negative Crack Width Determination)
Flexural Cracking in Concrete Structures EDWARD G. NAWY The state-of-the-art in the evaluation of the flexural crack width development and crack control of macrocracks is described. It is based on extensive research over the past 50 years in the United States and overseas in the area of macrocracking in reinforced.
- In Figure 2, crack width is a primary function of the de formation of reinforcement between adjacent Cracks 1 and 2, if the small concrete strain along crack interval ac is neglected. The crack width would hence be a function of the crack spacing, and vice versa, up to the level of stabilization of crack spacing (Figure 3).
- Crack Width Calculations 4 Design: general 4.1 Limit state requirements 4.1.1 Serviceability limit states 4.1.1.1 Cracking. Cracking of concrete should not adversely affect the appearance or durability of the structure.
- ACI 318 also indicates that the crack width is inher- ently subject to wide scatter (ACI 224R-01 indicates a coefficient of variation of 40%) and is influenced by shrinkage and temperature. In addition to cracks due to service loads, cracks also result from restrained shrinkage and thermal contrac- tion.
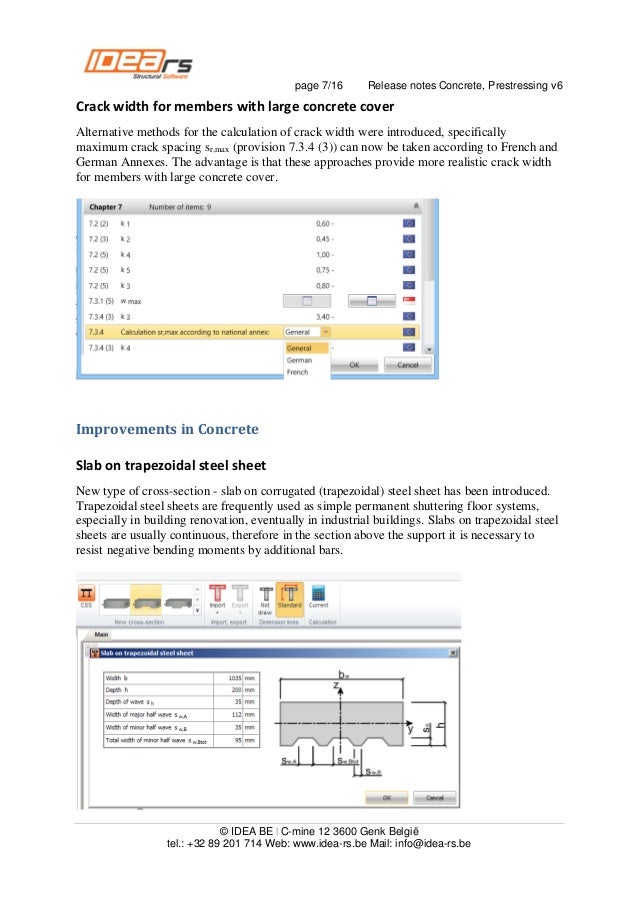
Eurocode 2 part 1-1: Design of concrete structures 7.3 Crack control
The crack width, wk, may be calculated as follows:
| wk = sr,max⋅(εsm - εcm) | (7.8) |
where:
- sr,max
- is the maximum crack spacing
- εsm
- is the mean strain in the reinforcement under the relevant combination of loads, including the effect of imposed deformations and taking into account the effects of tension stiffening
- εcm
- is the mean strain in the concrete between cracks.
| (7.9) |
where:

see application for a rectangular section or application for a T-section
with
- Ecm
- the secant modulus of elasticity of concrete
fct,eff = fctm or lower, (fctm(t)), if cracking is expected earlier than 28 days
| = (As + ξ1⋅A'p)/Ac,eff | (7.10) |
with
| ξ1 = | (7.5) |
with
- ξ
- the ratio of bond strength of prestressing and reinforcing steel, according to Table 6.2
- ΦS
- the largest bar diameter of the reinforcing steel
- ΦP
- the diameter or equivalent diameter of prestressing steel:
Φp = 1,6⋅√AP for bundles, where AP is the area of a prestressing steel,
Φp = 1,75⋅Φwire for single 7 wire strands,
Φp = 1,20⋅Φwire for single 3 wire strands, where Φwire is the wire diameter.
Pci Crack Width Calculation
kt = 0,6 for short term loading,
kt = 0,4 for long term loading.
• Where the bonded reinforcenlent is fixed at reasonably close centres within the tension zone (spacing ≤ 5(c + Φ/2), cf. Figure 7.2), the maximum crack spacing sr,max may be calculated as follows:
| sr,max = k3c + k1k2k4Φ / ρp,eff | (7.11) |
where:
Concrete Crack Width Calculation
k1 = 0,8 for high bond bars,
k1 = 1,6 for bars with an effectively plain surface (e.g. prestressing tendons).
k2 = 0,5 for bending,
k2 = 1,0 for pure tension.
Intermediate values of k2
Crack Width Calculation For Piles
should be used for cases of eccentric tension or for local areas:| k2 = (ε1 + ε2)/(2ε1) | (7.13) |
where ε1 is the greater and ε2 is the lesser tensile strain at the boundaries of the section considered, assessed on the basis of a cracked section.
• Where the spacing of the bonded reinforcement exceeds 5(c + Φ/2) (cf. Figure 7.2), or where there is no bonded reinforcement within the tension zone, the maximum crack spacing sr,max may be calculated as follows:
| sr,max = 1,3(h - x) | (7.14) |
where:
- h
- is the overall depth of the section (see Figure 7.1)
- x
- is the neutral axis depth of the section (see Figure 7.1).
This application calculates the crack width wk from your inputs. Intermediate results will also be given.
First, change the following option if necessary: